>
Time management is very much important in IIT JAM. The eduncle test series for IIT JAM Mathematical Statistics helped me a lot in this portion. I am very thankful to the test series I bought from eduncle.
Nilanjan Bhowmick AIR 3, CSIR NET (Earth Science)
Divam Kumar posted an Question
December 01, 2020 • 20:08 pm
![]()
30 points
30 points
- IIT JAM
- Mathematics (MA)
64. if (x, y) has an extreme value at (a, b), then: (a) ,(a,b) > 1 (b) 1,(a, b) <2 (c) ,a, b) + 0 (d) s,(a, b) = 0 0. it a functionf is continuous on ja, b]. th
64. If (x, y) has an extreme value at (a, b), then: (A) ,(a,b) > 1 (B) 1,(a, b) <2 (C) ,a, b) + 0 (D) S,(a, b) = 0 0. IT a functionf is continuous on Ja, b]. then by first meant value theorem there exists a number e [a, b] such that: (A)fdr > f)6-) (B) fd < f)(6 - a) (C)fd =l+f(E) (6 -a) (D)fd = f)(6- a) 66. A function f 1s bounded and integrable functions on a, b] and there exists a function F such that F'=fon [a, b], then: (A) fdr = F(6) - F(a) (B) d = F(6) + F(a) (C) fd F(6) - F(a) (D) None of these 67. If a sequence Jn converges to f uniformly on la, b], and each function Jn is integrable on la, b], then : (A)Sdt = - lim,-.S,d, Vx e [a, b] (B)fdt = lim,--J, d, VxE [a,b] (C) dt lim, , Jn di, Vxe [a, b] (D) None of these
1 Answer(s)
Answer Now
- 0 Likes
- 2 Comments
- 0 Shares
Do You Want Better RANK in Your Exam?
Start Your Preparations with Eduncle’s FREE Study Material
- Updated Syllabus, Paper Pattern & Full Exam Details
- Sample Theory of Most Important Topic
- Model Test Paper with Detailed Solutions
- Last 5 Years Question Papers & Answers
Sign Up to Download FREE Study Material Worth Rs. 500/-
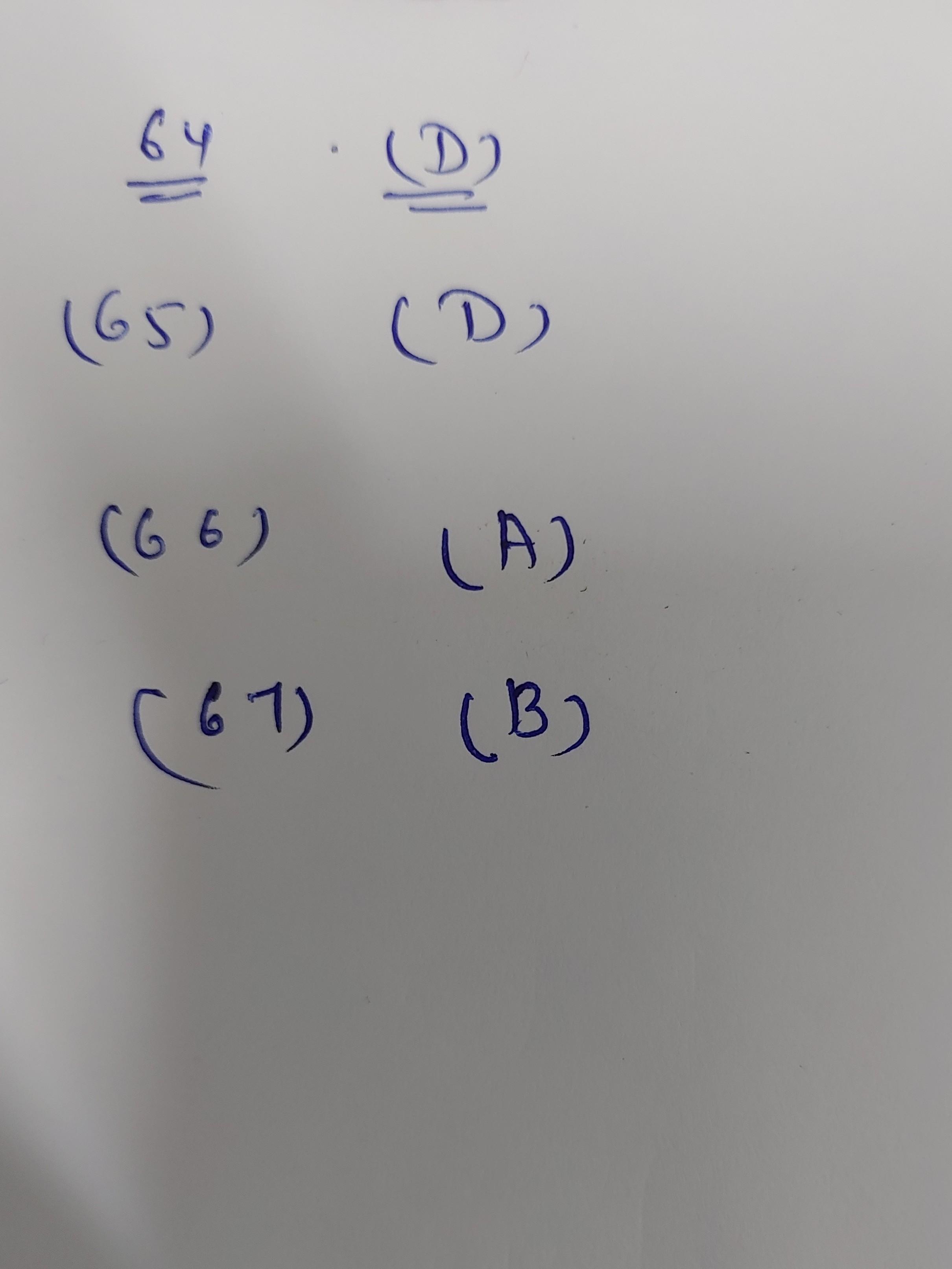
Divam kumar
thanks